Abstract

This study is concerned with the development of a high-order numerical model to solve incompressible water wave motion based on improved nonlinear dispersive Boussinesq equations. A third-order Adams-Bashforth and a fourth-order Adams-Mouton predictor-corrector scheme was selected in an attempt to eliminate the truncation error terms that would be of the same form as the dispersive terms in the Boussinesq equations with second order schemes as in many other studies.

Eddy viscosity type momentum correction term was added into the Boussinesq equation to simulate the energy loss due to wave breaking and to extend the model application to surf zone wave transformation. The location of the breaking point was determined through a wave breaking criterion using the ratio of horizontal water particle velocity to wave celerity.

A moving boundary technique utilizing linear extrapolation is developed to investigate wave runup and rundown. Wave absorption at an open boundary was simulated by solving the Sommerfeld radiation and introducing sponge layer into the model.

Breaking regular wave runup propagation on a sloping beach is simulated. It would seem that inclusion of an accurate dissipation term becomes increasingly important with increasing degree of wave breaking. In regard to breaking regular wave runup simulation, additional dissipation term acted as bottom friction was required for long term stability in surf zone area. The validity of the model was confirmed by comparing computations with analytical solutions and measured data.

Introduction

Coastal zone is one of the most valuable regions on earth from the viewpoint of the ecosystem and human welfare. The coastal zone has been recognized as natural resources for the activities of human beings and has been utilized for various purposes. Coastal zone has also an important ecological value due to plenty of diversity in habitats.

These days the protection of coastal areas has become one of the more pressing environmental problems in many countries. Most of these problems are related to the wave and current phenomena in the nearshore zone. These phenomena have thus attracted immensely the interest of engineers in order to safeguard multi-faceted activities in the coastal zone.

Understanding nearshore waves has become an indispensable tool in the estimation of forces for the proper design and construction of coastal infrastructures. Waves attack coastal structure and infiltrate harbor entrance, generating disturbance in the sheltered waters. Hence, it is also necessary to know beforehand the magnitude and direction of propagating waves to design the optimal layout of harbors.

As waves propagate toward shore, a combination of shoaling, refraction, reflection, diffraction, and breaking effects modify the waveform, and the wave characteristics will be very different from those in deep water. The ability to accurately predict wave transformation from deep to shallow water is vital to an understanding of coastal processes. By the refraction the directions of wave propagation as well as the wave height vary in accordance with the sea bottom topography. By the wave shoaling the wave height gradually change as a result of the change of the rate of energy flux due to the reduction in water depth, even if no refraction takes place.

When incoming waves are interrupted by a barrier such as a breakwater or an island, the waves curve around the barrier and penetrate into the sheltered area, this phenomenon is called diffraction. When waves reach a water of depth less than a few times the wave height, waves begin to break and the overall wave height decreases as the wave energy is dissipated.

The processes of wave breaking, runup, setdown and setup of the mean water level, turbulent energy production, generation of nearshore currents and generation of infragravity waves are important driving mechanisms for the transport of sediments and pollutants as well as erosions in coastal regions.

Most coastal erosion and accretion problems can be prevented or mitigated with some engineering knowledge of coastal sedimentation. Periodic dredging operation and channel navigation can be planned and managed more precisely and effectively. The understanding of effects caused by existing or newly constructed coastal structure is also some of the benefits derived from a detailed modeling of the wave and current phenomena in shallow water.

The effects of man-made or naturally caused changes around coastal area can be examined through physical modeling. This usually involves intricate and time consuming setting up of a scaled-down physical model and requires accurate measurements of wave data. With the availability of advanced computing facilities, numerical model has become an attractive alternative. The shift towards numerical modeling has resulted in rapid developments of numerical methods for solving water wave equations.

The greatest advance of numerical model over a physical model is its ability to determine the required information at a much shorter period with a comparatively larger degree of accuracy. The same numerical model may also be used repeatedly on different problems with only some minor modification in boundary conditions, bathymetry and incident wave condition.

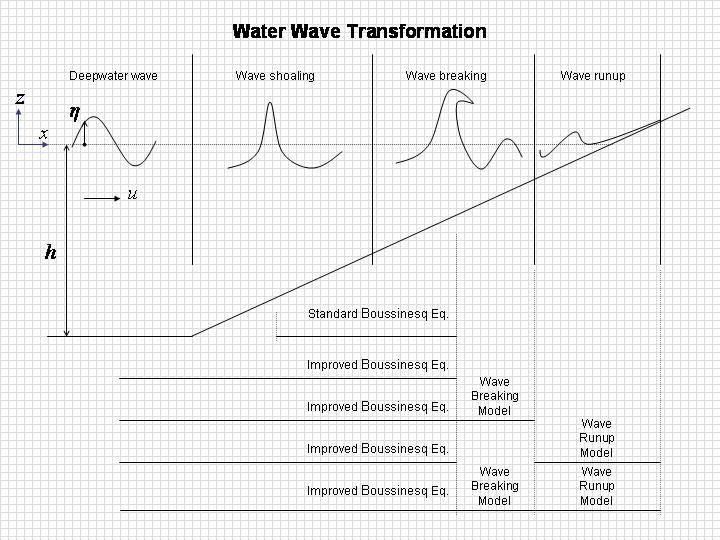
It has long been a goal of coastal engineers to produce a computational wave model that is capable of accurately simulating wave motion from deep water through the surf zone. To do this, a model would have to include, among other things, nonlinear shoaling, refraction, diffraction, wave-wave interaction, breaking, and runup.

Early numerical model, owing perhaps to limitations in computing capability, were mostly based on the Airy (1845) linear wave theory wherein the pressure distribution in the vertical is assumed to be hydrostatic.

In the nearshore region, where water depth and shallow and amplitude may still be large, wave is highly nonlinear, characterized by asymmetric orbital motion of the water particle. The nonlinearity becomes increasingly dominant with decreasing water depth. Hence, it may be expected that numerical models based on the linear wave theory will not provide an accurate simulation of the nearshore wave phenomenon.

Linear theory also cannot simulate the secondary effects of the wave action such wave set-up and wave set-down. Nonlinear wave theories include the wave nonlinearities and simulate simultaneously the wave phenomenom and its secondary effects.

The mathematical description on nonlinear water wave involves the Laplace equation combined with nonlinear boundary conditions at the free-surface and at the sea bottom. This problem is complicated by the fact that the moving surface boundary is part of the solution. Direct numerical methods for solving the full equations exist but they are extremely time consuming and can only be applied to small scale problems. As it is currently impractical to perform a full solution over any significant domain, approximate models must be used. For this reason there is a strong interest in so-called Boussinesq-type formulations of the water wave problem.

The principle behind Boussinesq formulations is to incorporate the effects of non-hydrostatic pressure, while eliminating the vertical coordinate, thus significantly reducing the computational effort relative to a full three-dimensional solution. This principle was initially introduced by Boussinesq (1872), who derived new governing equations under the assumption that the magnitude of the vertical velocity increases polynomially from the bottom to the free surface which inevitably leads to some form of depth limitation in the accuracy of the embedded dispersive and nonlinear properties. Hence, Boussinesq-type equations are conventionally associated with relatively shallow water.

Due to increasing error in the modeled linear dispersion relation with increasing water depth, the standard Boussinesq equations are limited to relatively shallow water. Recently, efforts were made by a number of investigators to derive alternative forms of Boussinesq equations that can be applied in deeper water regions. In order to make the Boussinesq equations applicable in deeper water, many researchers have suggested the ways to extend the validity range of the equations. The extended Boussinesq equations normally have adjustable polynomial approximations for the exact dispersion relationship, a major improvement from the standard ones.

Recent developments in numerical schemes for solving Boussinesq-type equation have placed immense interest in nonlinear dispersive wave models based on the Boussinesq equations. One set of candidate models is the various Boussinesq-type equations (Peregrine 1967; Madsen and Sørensen 1992; Nwogu 1993; Wei et al. 1995). These can describe, to varying degrees of accuracy in representing nonlinearity and dispersion, most phenomena exhibited by non-breaking waves in finite depths.

However, as derived, they do not include dissipation due to wave breaking and thus become invalid in the surf zone. Therefore, it is necessary to implement the proper wave breaking model to the well developed Boussinesq wave model for simulating the surf zone phenomena in nearshore zone.

Although all of these efforts extend the usage of the Boussinesq-type equation model such that wave evolution from relatively deep water to the breaking point could be accurately captured, but the process of runup and rundown is not. There has been only limited success developing a general model which can theoretically predict the runup of arbitrary wave especially the wave break during approach to the shoreline. Even non-breaking waves are difficult to treat because the shoreline represents a moving boundary, which must be computed as part of the solution.

Objectives of the Study

The main objective of this study is to develop high-order numerical scheme that can be used to solve the improved nonlinear dispersive one-dimensional Boussinesq equations. This scheme has ability to accurately predict wave transformation from deep to shallow water and to simulate most of the phenomena of interest in the nearshore zone.

The numerical model based on these equations is also extended to simulate one-dimensional wave propagation in the shallow water region over a horizontal or mildly sloping bed to include wave transformation inside the surf zone.

A one-dimensional wave breaking criterion is used to determine the location of the breaking point. With the determination of the breaking point, by choosing a proper wave breaking model and implement it in the developed wave model, simulation of the surf zone wave transformation as well as secondary effects like wave set-down and wave set-up are also simulated.

Finally, a moving boundary technique utilizes linear extrapolation is developed to investigate wave runup and rundown. The moving boundary is tested for accuracy using one-dimensional analytical solutions.

References can be found here.

Boussinesq-type Equations

Boussinesq-type equations are capable of providing accurate description of wave evolution in coastal regions. The onset of recent developments in the field of Boussinesq models was triggered by two events. The first was the increasing availability of the computer resources needed to run the models. The second was the development of variants of the theory which could be optimized to obtain better dispersion properties at larger values, thus allowing the model to treat a larger range of water depths.

The earliest depth-averaged model that included both weakly dispersive and nonlinear effects was derived by Boussinesq in 1871. The equations were derived for horizontal bottoms only. Later, Mei and LeMehaute (1966) and Peregrine (1967) derived Boussinesq equations for variable depth. Mei and LeMehaute (1966) used the velocity at the bottom as the dependent variable, whereas Peregrine (1967) used the depth-averaged velocity.

The past decade saw the advent and widespread applications of Boussinesq-type equation models for studying wave propagation. Due to the wide popularity in the coastal engineering community, the equations derived by Peregrine (1967) are often referred to as the standard Boussinesq equations, which are able to describe the nonlinear transformation of irregular and multidirectional waves in shallow water. The vertical velocity is assumed to vary linearly over the depth.

The Boussinesq equations include the lowest-order effects of frequency dispersion and nonlinearity. They can thus account for the transfer of energy between different frequency components, changes in the shape of the individual waves and the evolution of wave groups, in the shoaling irregular wave train (e.g., Freilich and Guza 1984).

However, the standard Boussinesq equations (Peregrine, 1967) had two major limitations that they are only applicable to relatively shallow water depths:
(1) The depth-averaged model poorly described the frequency dispersion of wave propagation in intermediate depths.
(2) the weakly nonlinear assumption limited the largest wave height that could accurately be modeled.
To keep errors in the phase velocity less than 5%, the water depth has to be less than about one-fifth of the equivalent deep-water wavelength (McCowan 1987).

Recently, a number of attempts have been made to extend the range of applicability of the equations to deeper water by improving the dispersion characteristic of the equations. Witting (1984) used a different form of the exact, fully nonlinear, depth-integrated momentum equation for one horizontal dimension, expressed in terms of the velocity at free surface. A Taylor-series-type expansion was used to relate the different velocity variables in the governing equations, with the coefficients of the expansion determined to yield the best linear dispersion characteristics. By retaining terms up to the fourth order in dispersion, Witting obtained relatively accurate result for both deep and shallow water waves. However, the expansions presented by Witting are only valid in water of constant depth.

Murray (1989) and Madsen et al. (1991) examined the dispersion properties of various forms of the Boussinesq equations as well as Witting’s (1984) Pade approximation of the linear dispersion relation for Airy waves. Based on the excellent characteristic of Pade approximant, the writers have introduced an additional third-order term to the momentum equation to improve the dispersion properties of the Boussinesq equations. The third-order is derived from the long wave equations and reduces to zero in shallow water, resulting in the standard form of the equations for shallow water. The equations assume a constant water depth and, thus, are not applicable to shoaling waves.

On the other hand, by defining the dependent variable as the velocity at an arbitrary depth, Nwogu (1993) achieved a rational polynomial approximation to the exact linear dispersion relationship without the need to add higher order terms to the equations. Although the arbitrary location could be chosen to give a Pade approximation to the linear dispersion relationship, Nwogu (1993) chose an alternative value which minimized the error in the linear phase speed over certain depth range.

Both Madsen et al’s and Nwogu’s expressions give more accurate dispersion relation in intermediate water depths than the standard Boussinesq equations do. They have shown by examples that the extended equations are able to simulate wave propagation from much deeper water to shallow water. Of these two models, the Boussinesq equations derived by Nwogu (1993) are obtained through a consistent derivation from the continuity and Euler equations of motion.

Since Nwogu’s extended Boussinesq equations were proposed, it quickly became one of the most cited works in the field of water wave modeling by using Boussinesq equations. The popularity of Nwogu’s equations among coastal modelers is due to the relatively simple form of the equations as well as the balanced ability of representing wave nonlinearity and dispersion.

Liu (1994) and Wei et al. (1995) presented a set of highly nonlinear Boussinesq-type equations that not only can be applied to intermediate water depth but also are capable of simulating wave propagation with strong nonlinear interaction. Wei et al. (1995) have also developed a high-order numerical scheme to solve these equations.

Although the higher-order Boussinesq equations for the improvement of the nonlinear and dispersive properties in water waves have been attempted and successful in certain aspects, most of them involved numerous additional derivatives and hence made the accurate numerical solution increasingly difficult to obtain. For this reason, Nwogu’s extended Boussinesq equations remain to be a good choice for coastal wave modeling, especially for engineering applications.

References can be found here.